Research Projects

Multiphysics waveform tomography in complex/unknown environments

with: Jian Song and Yang Xu

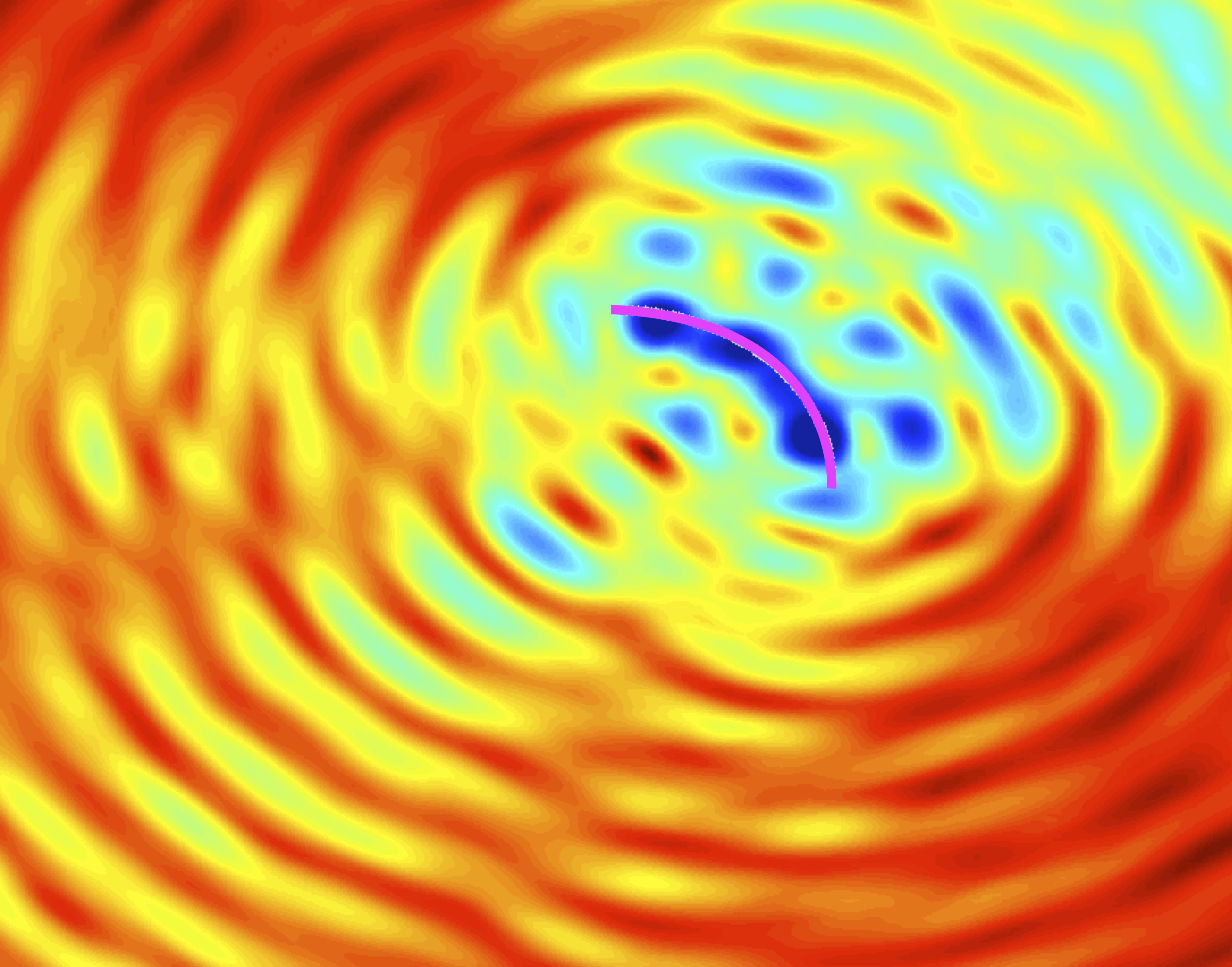
This research aims to establish a holistic data inversion framework for the reconstruction and characterization of evolving processes in environments with a priori unknown structure and material properties. This is accomplished by building on recent advances in inverse scattering and deep learning. The main idea is to design physics informed and mathematically sound cost functions which allow for fast (yet proper) processing of large and noisy data sets.

Data-driven design of materials

with: Noah Francis

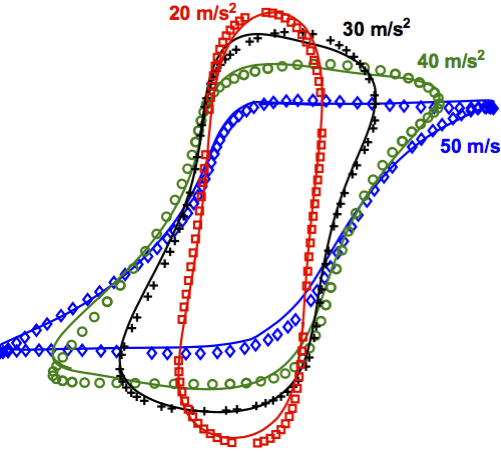
This work aims to develop a systematic platform for composing new materials/components (of random/periodic microstructure) with specific wave guiding characteristics. Our current focus is primarily on a class of generalized continua with the potential of achieving extreme properties. In this vein, asymptotic analysis meets deep neural networks to generate efficient (yet accurate) maps between the design parameters and effective properties (or dispersive behavior) of interest.

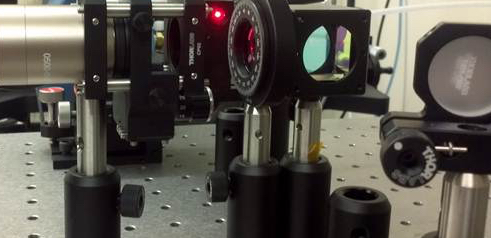
Laser ultrasonics

with: Jian Song, Abigail Schmid, and Noah Francis

This research aims to generate new experimental concepts for (a) in-situ implementation of the next-generation imaging solutions pertinent to complex environments, (b) validation of new data-driven techniques for multiscale modeling of composites, and (c) examination of newly designed materials and structures at the laboratory scale. To this end, we primarily make use of the laser-based sensing technology thanks to its versatility and broad dynamic range.

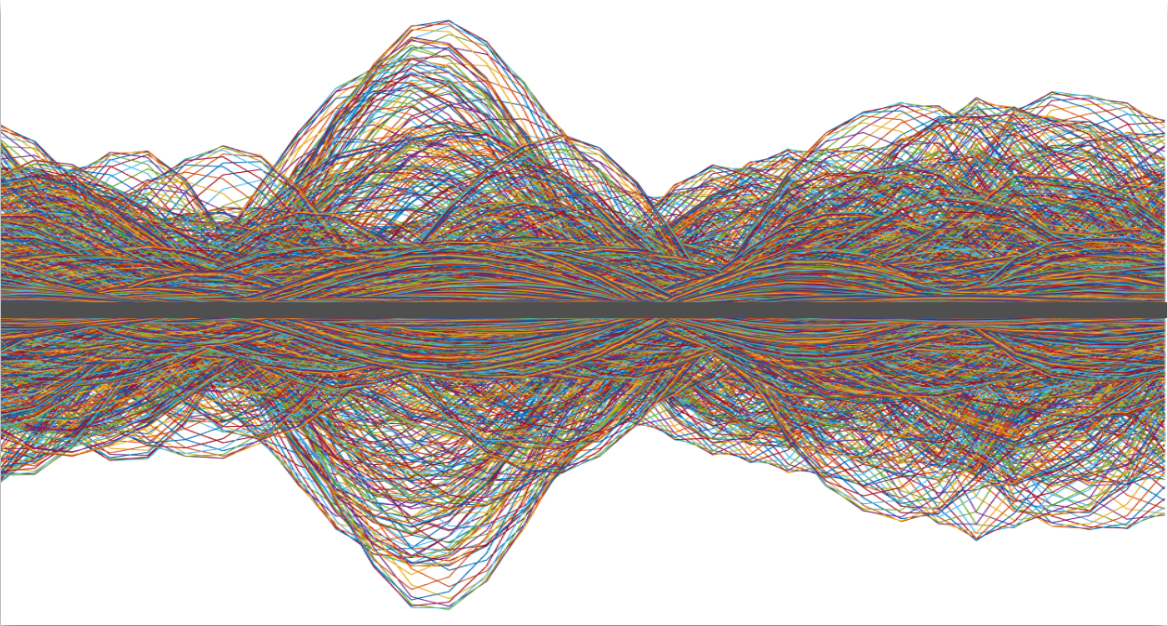
Wave-based bayesian characterization of complex materials

with: Abigail Schmid

This project is focused on application of bayesian inference to analyze broadband full-waveform measurements for better understanding of the multiscale mechanics of composite materials with random microstructure. In this vein, recent methods in data-driven modeling by artificial intelligence are deployed. Model-free data inversion schemes, in particular, entail differentiation and inversion of noisy data which require appropriate uncertainty quantification of thus-obtained solutions.

A holistic approach to imaging heterogeneous fractures

with: Bojan Guzina and Houssem Haddar

The goal of this study is two-fold: i) a robust geometric reconstruction of fractures from the scattered field data using a carefully designed indicator functional that features low sensitivity to measurement noise and imposes no major restrictions on the illumination frequency or the sensing configuration, and ii) identification of the fracture's heterogeneous boundary condition. This is accomplished via an extension of the so-called generalized linear sampling method (GLSM), and the use of a boundary integral equation (with known geometry) for a fracture surface with elastic contact condition.

Wave propagation through a rough interface: an experimental study

with: Bojan Guzina and Roman Tokmashev

This study takes the first step toward experimental verification of the proposed hybrid method for "imaging" the true contact law along the interface of a (stationary or propagating) heterogeneous fracture. To this end, slab-like laboratory specimens are: a) damaged, b) subjected to suitable static stress, and c) excited by O(10 - 100 kHz) ultrasonic waves, while monitoring the induced surface motion over a high density of sensing points - in a vicinity of the fracture, via a Scanning Laser Doppler Vibrometer (SLDV) system capable of tracking triaxial particle velocity with nanometer accuracy and millimeter spatial resolution. By invoking the concept of elastography (previously developed for the purposes of medical imaging) and performing comprehensive signal processing of the SLDV data, we aim to recover i) the (spatially-varying) displacement jump across the fracture surface, and ii) the affiliated traction vector. This enables point-wise characterization of the fracture's interfacial contact condition by revealing the underpinning traction-displacement jump relationship and its evolution with time.

High-frequency inverse scattering via Topological Sensitivity

with: Bojan Guzina

In the context of acoustic inverse scattering, this study deciphers the topological sensitivity (TS) as a tool for simultaneous shape reconstruction and characterization of impenetrable objects in the high-frequency regime. It is assumed that the anomaly is simply connected and convex, and that the measurements of the scattered field are of the far-field type. In this setting, the formula for TS - which quantifies the perturbation of a cost functional due to a point-like impenetrable scatterer - is expressed as a pair of nested surface integrals: one taken over the boundary of a hidden obstacle, and the other over the measurement surface. By employing the multipole expansion method, Kirchhoff approximation, asymptotic analysis of diffraction integrals, and catastrophe theory, the TS is found to survive upon three asymptotic lynchpins, namely (i) the near-boundary approximation for sampling points close to the ‘exposed’ surface of an obstacle; (ii) uniform expansions synthesizing the diffraction catastrophes for sampling points near caustic surfaces, lines and points; and (iii) stationary phase approximation. It is then shown that, in the case of the full source aperture, the TS is asymptotically dominated by the (explicit) near-boundary term featuring shape reconstruction capability. The analysis further shows that, when the character of an anomaly is unknown beforehand, the latter can be effectively exposed via the sign of the leading term inside the reconstruction.

On the elastic-wave imaging of fractures with interfacial stiffness

with: Bojan Guzina

The TS concept is extended to enable simultaneous 3D reconstruction of fractures and qualitative characterization of their interface, in low-frequency regimes, by way of elastic waves. Interactions between the two surfaces of a fracture, due to e.g. presence of asperities, fluid, or proppant, are described via the linear slip model. The proposed TS sensing platform entails point-wise interrogation of the subsurface volume by infinitesimal fissures endowed with interfacial stiffness. It is found that irrespective of fracture boundary condition, the TS is capable of reconstructing its location and identifying the normal vector to the fracture surface without iterations. Assuming “low frequency” illumination, it is further shown that by certain choices of the trial fracture stiffnesses, the fracture interfacial condition can be qualitatively identified at virtually no surcharge. The proposed developments are integrated into a computational platform based on a regularized boundary integral equation (BIE) method for 3D elastodynamics, and illustrated via a set of canonical numerical experiments.

Experimental reconstruction of Nonlinear Normal Modes

with: Hamid Ahmadian

The concept of Nonlinear Normal Modes (NNMs) used as a powerful tool for extending the classical modal analysis to nonlinear systems. This study proposes two feasible ways to reconstruct NNMs from experimental data: i) the equivalent linear model for a nonlinear system is formulated - in terms of the amplitude of motion in a vicinity of its resonance frequencies, whereby the affiliated NNMs are identified as the normal modes of those equivalent systems, and ii) the motion of a nonlinear system near a resonance frequency is taken as its corresponding NNM. The later is capable of recovering NNMs of complex structures with global nonlinearities that requires no knowledge of the system nonlinearity a priori, and thus, is suitable for its accurate characterization. In an experimental case study featuring a beam structure with frictional contact support endowed with micro-slip, both methods are implemented and shown to produce similar results.

Nonlinear model identification of frictional interfaces under micro-vibro- impact and slip

with: Hamid Ahmadian and Hassan Jalali

This study investigates the nonlinear behavior of a frictional contact in situations where micro-impacts develop in the normal direction to the interface. In particular, the effect of (temporally) variable normal load on the frictional forces are studied. As a result, a new interface law is proposed that is capable of taking into account such effect. In an experimental case study, the contact is excited in a dual-harmonic fashion, catering for a reliable dual-mode identification procedure. Using measured data and normal modes of the affiliated equivalent linear systems, suitable NNMs are obtained for the purpose of representing the system response. The resulting reduced order model is representative of dominant nonlinear effects in the contact area. The force state mapping is then invoked to identify the contact restoring forces (in both normal and tangential directions) in time domain. The latter is used to determine the parameters of a new friction law developed in the spirit of the Valanis model. It is shown that the model is capable of predicting the main nonlinear characteristics of the contact interface and regenerates the experimental results at different vibration amplitudes.